All data in computer programs is internally stored as bits, i.e., as numbers 0 and 1. It turns out that there are many uses for bit manipulation in competitive programming.
In programming, an n bit integer is internally stored as a binary number that consists of n bits. For example, the C++ type int is a 32-bit type, which means that every int number consists of 32 bits. For exmaple, the bit representation of the int number 42 is 00000000000000000000000000101010.
There are several bit operations supported by a typical ALU, some of them being AND, OR, NOT, XOR, and bit shifts
n&1 = 1 implies n is odd, and n&1 = 0 implies n is even. This executes much faster than the typical way to check if a number is odd (ie. n%2 = 1).1<<k gives 2^k. This makes it easy to check if the kth bit of a number from the right is set or not, ie. n&(1<<k) == 1. This can be used to easily convert a decimal number to binary as follows:
C++
for (int i = 31; i >= 0; i--){
if (n&(1<<i)) cout << "1";
else cout << "0";
}x |= (1 << k) sets the k th bit of x to one, the formula x &= ~(1 << k) sets the k th bit of x to zero, and the formula x ^= (1 << k) inverts the k th bit of x.x &= (x − 1) sets the last one bit of x to zero, and the formula x &= − x sets all the one bits to zero, except for the last one bit. The formula x |= (x − 1) inverts all the bits after the last one bit. Also note that a positive number x is a power of two exactly when x & (x − 1) = 0, and x&(-x) gives the highest power of 2 which divides x.The g++ compiler provides the following functions for counting bits:
1. __builtin_clz(x): the number of zeros at the beginning of the number
2. __builtin_ctz(x): the number of zeros at the end of the number
3. __builtin_popcount(x): the number of ones in the number
4. __builtin_parity(x): the parity (even or odd) of the number of ones
Consider the following problem: Given an $N * N$ grid whose each square is either black (1) or white (0), calculate the number of subgrids whose all corners are black. For example, the grid
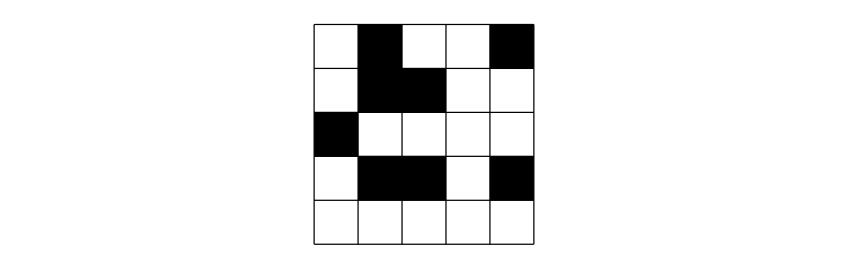
contains two such subgrids:
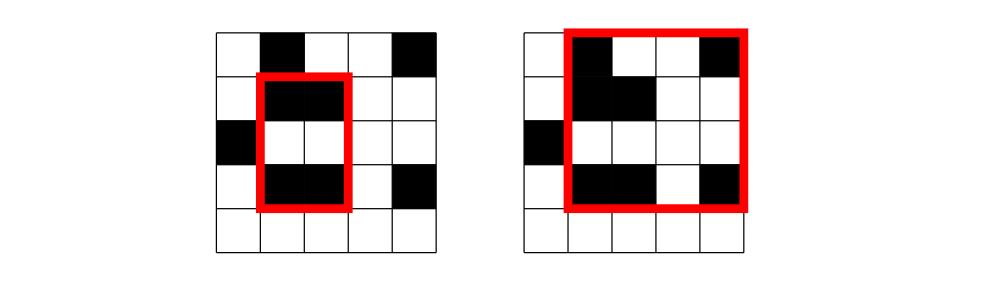
There is an $O(n^3)$ time algorithm for solving the problem: go through all $O(n^2)$ pairs of rows and for each pair $(a, b)$ calculate the number of columns that contain a black square in both rows in $O(n)$ time. The following code assumes that $color[y][x]$ denotes the color in row y and column x :
int count = 0;
for (int i = 0; i < n; i++) {
if (color[a][i] == 1 && color[b][i] == 1) count++;
}
Then, those columns account for count*(count − 1)/2 subgrids with black corners, because we can choose any two of them to form a subgrid. To optimize this algorithm, we divide the grid into blocks of columns such that each block consists of N consecutive columns. Then, each row is stored as a list of N-bit numbers that describe the colors of the squares. Now we can process N columns at the same time using bit operations. In the following code, $color[y][k]$ represents a block of N colors as bits.
int count = 0;
for (int i = 0; i <= n/N; i++) {
count += __builtin_popcount(color[a][i]&color[b][i]);
}
The resulting algorithm works in $O(n^3/N)$ time.
A comparison was done for a randomly generated grid of size 2500 × 2500 between the original and bit optimized implementation. While the original code took 29.6 seconds, the bit optimized version only took 3.1 seconds with N = 32 (int numbers) and 1.7 seconds with N = 64 (long long numbers).
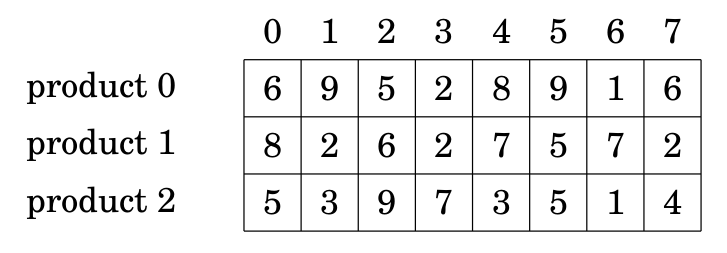
Consider the following problem: We are given the prices of k products over n days, and we want to buy each product exactly once. However, we are allowed to buy at most one product in a day. What is the minimum total price? For example, consider the following scenario ( k = 3 and n = 8):
In this scenario, the minimum total price is 5:
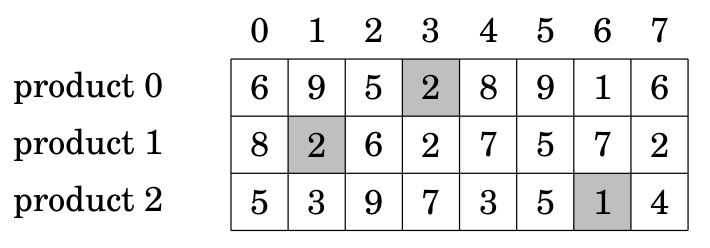
Let $price[x][d]$ denote the price of product x on day d. For example, in the above scenario $price[2][3]$ = $7$. Then, let total(S, d) denote the minimum total price to buy all products by day d, when a subset S has already been bought. Using this function, the solution to the problem is total({}, n − 1).
To represent the subset S, we use bit masks. Consider a 32-bit integer N. If the kth bit (from the right) of N is set, then the kth product is a part of the subset, else not.
Therefore on each day, we have a choice to buy one of the products which hasn't been bought before or buy no product. The base conditions would be: - If all products have been bought, then return 0 - If we have exceeded the total number of days return INF.
Therefore the C++ code is as follows:
int total(int mask, int day){
if(mask == ((1<<k) -1)) //All products have been bought.
return 0;
if(day == -1)
return INF;
int ans = INF;
for(int i=0; i<k; i++)
if(mask & (1<<i) == 0) //Product i hasn't been bought yet.
ans = min(ans, total(mask | (1<<i), day-1) + price[i][day]);
//Not buying anything
ans = min(ans, total(mask, day -1));
return ans;
}
Optimizing the above code by memoizing:
int dp[1<<k][n]; //Initially all values set to -1
int total(int mask, int day){
if(mask == ((1<<k) -1))
return 0;
if(day == -1)
return INF;
if(dp[mask][day] != -1)
return dp[mask][day];
int ans = INF;
for(int i=0; i<k; i++)
if(mask & (1<<i) == 0)
ans = min(ans, total(mask | (1<<i), day-1) + price[i][day]);
ans = min(ans, total(mask, day -1));
return dp[mask][day] = ans;
}
The above code runs in $O(nk2^k)$.